In collaboration with Rob Schoelkopf’s group, we have theoretically proposed and experimentally demonstrated the key ingredients of universal control of an oscillator with dispersive coupling to a qubit.
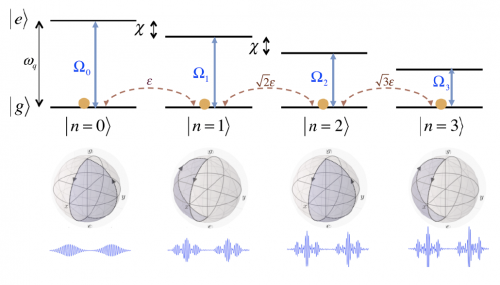
We investigate quantum control of an oscillator mode off-resonantly coupled to an ancillary qubit. In the strong dispersive regime, we may drive the qubit conditioned on the number states of the oscillator, which, together with displacement operations, can achieve universal control of the oscillator. Based on our proof of universal control, we provide a straightforward recipe to perform arbitrary unitary operations on the oscillator. This universal control scheme of the oscillator enables us to efficiently manipulate the quantum information stored in the oscillator, which can be implemented using superconducting circuits. [PRA 92, 040303 (2015)]
Experimentally, we show that the SNAP gate allows control over the quantum phases by correcting the unwanted phase evolution due to the Kerr effect. Furthermore, by combining the SNAP gate with oscillator displacements, we create a one-photon Fock state with high fidelity. Using just these two controls, one can construct arbitrary unitary operations, offering a scalable route to performing logical manipulations on oscillator encoded qubits. [PRL 115, 137002 (2016)]